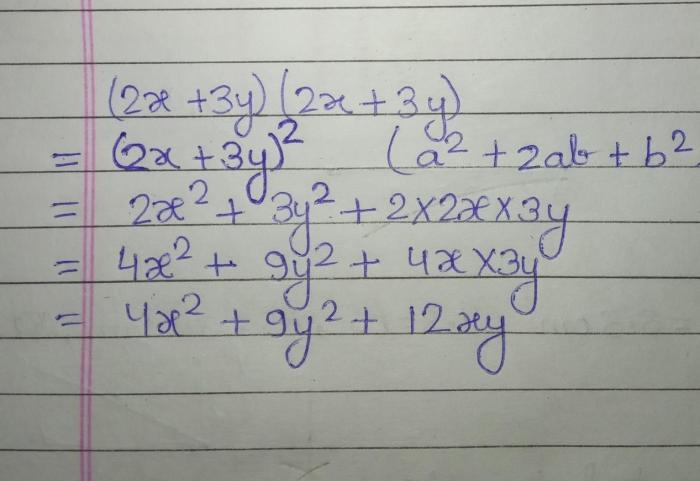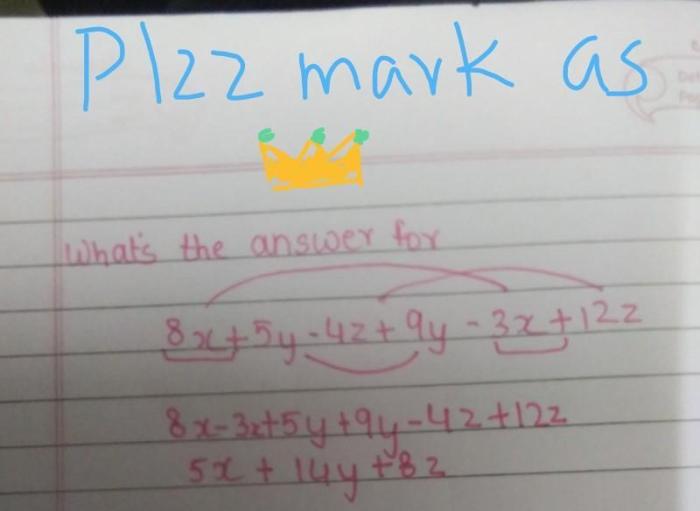Solve the system by elimination x 5y 4z 10 – Solve the system by elimination x + 5y + 4z = 10 embarks on an enlightening journey into the realm of linear equations, where we delve into the intricacies of solving systems using the elimination method. This technique, a cornerstone of algebra, empowers us to unravel complex systems of equations, revealing the hidden relationships between variables and their coefficients.
Our exploration begins with a thorough examination of the system’s variables and their interdependence. We establish the groundwork for understanding how these variables interact, paving the way for skillful manipulation of equations. Step-by-step, we unravel the process of isolating variables, employing substitution to determine the values of the remaining variables.
Elimination Method for Solving Systems of Equations

In mathematics, systems of linear equations arise in various applications. The elimination method is a fundamental technique for solving such systems, involving the manipulation of equations to eliminate variables and find the values of the remaining variables.
Variable Identification
In a system of equations, variables represent unknown quantities. In the given system x + 5y + 4z = 10, the variables x, y, and z represent the unknown values that we seek to determine. The coefficients 5 and 4 represent the multiplicative factors of the variables y and z, respectively.
Equation Manipulation
To solve the system, we manipulate the equations to isolate one variable. For example, we can subtract 5y from both sides of the first equation to get x + 4z = 10 – 5y. This allows us to solve for x in terms of y and z.
Elimination Method, Solve the system by elimination x 5y 4z 10
The elimination method involves multiplying equations by suitable constants and adding or subtracting them to eliminate a variable. For instance, if we multiply the first equation by 5 and subtract it from the second equation, we eliminate y:
- 5(x + 5y + 4z = 10) -> 5x + 25y + 20z = 50
- -(x + 5y + 4z = 10) -> -x – 5y – 4z = -10
- 4x + 20z = 60
Solution Verification
Once we have found the values of the variables, it is crucial to verify the solution by substituting them back into the original equations. This ensures that the values satisfy all the equations in the system.
Example Application
The elimination method finds applications in various fields, such as engineering, economics, and physics. For instance, in structural engineering, it can be used to determine the forces acting on a structure under different loading conditions.
Extension to Matrices
The elimination method can be extended to solve systems of linear equations represented by matrices. Matrices provide a systematic way to represent and manipulate systems of equations, simplifying the process and making it suitable for solving larger systems.
Quick FAQs: Solve The System By Elimination X 5y 4z 10
What is the elimination method?
The elimination method is a technique used to solve systems of equations by eliminating variables through addition or subtraction of equations.
How do I verify the solution of a system of equations?
To verify the solution, substitute the values of the variables back into the original equations and check if the equations hold true.
Can the elimination method be used to solve any system of equations?
The elimination method is most effective for systems of equations with two or three variables. For larger systems, matrix methods may be more efficient.